Rather than sporadically give information in posts... Let me take a moment to explain a few things with respect to aerodynamics, cD and a few terms that go with that.... Basically, I'm about to regurgitate some of the concepts you might learn in a fluid mechanics course (but minimizing the math component)
First, some definitions
Reynolds number: a ratio between the inertial forces (momentum of the working fluid) to viscous forces (property of resistance to shearing) | dependent on: body length, fluid velocity, fluid density, dynamic fluid viscosity, kinematic fluid viscosity.
Frictional Drag: this is the drag between the moving fluid and relativity stationary surface. May be referred to as viscous drag - I'll switch back of forth most likely. This value is dependent/sensitive on the Reynolds number.
Pressure Drag: this is the drag as a result of a pressure drop - wake. Think of that big blast of wind from a semi truck, or the waves generated by a boat. Pressure drag is mildly dependent on the Reynolds number, but generally not sensitive to it. Pressure drag is highly sensitive to body shape.
For both Frictional and Pressure drag.... It's important to note that they are both VERY dependent on fluid viscosity. That is, in inviscid (viscosity = 0) flow, both of drop to 0.
AoA: Angle of attack - draw a line in parallel to fluid flow. Now draw a line from the leading edge of a body to the trailing edge. Measure the angle between these two lines.
Streamlined Body: Drag losses are primarily due to viscous losses. Streamlined bodies of the same thickness are significantly more aerodynamic than a bluff body. Note that a streamlined shape doesn't necessarily mean a streamlined body - at a high AoA, flow separation causes great pressure drops.
Bluff Body: Drag losses at high Reynolds numbers are primarily due to momentum losses - meaning, pressure drop as a result of a wake.
Free Stream: This is the flow that is not impeded by a body.
Boundary Layer: I can spend a lot of time on this, but I'll abridge it... The boundary layer is the fluid directly beside the body. The general profile of the boundary looks something like this:

where the length of the lines represent a velocity vector - the longer the line, the faster the flow. At the point where the fluid meets the body, there is a
zero slip condition. That is, at this point the fluid
does not move. There are some important implications of this as it applies to differential calculus - but don't worry about that, just understand this concept

Below is the shape of the boundary layer (note that the y scale is increased for easier reading):

Note the several phases of the boundary layer:
- Laminar
- Transitional
- Turbulent
And just for fun - here's a real world example:
 Laminar Flow:
Laminar Flow: Fluid "layers" (streamlines) flow over each other in a parallel fashion smoothly over each other.
Transitional Flow: This is more of a mathematical zone as laminar turns into turbulent. I call it mathematical because there are specialized equations that apply to transitional flow only.
Turbulent Flow: The streamlines, instead of slipping over each other, diverge in chaotic eddies. Note that chaotic does not mean random, it "simply" means that it is
highly dependent on initial flow conditions - small changes in these conditions create great differences in output.
Please note, there is no critical distinction between laminar, transitional and turbulent flow. The mathematical distinction is almost arbitrary - it's just a number that the community has agreed to use

Okay, I think that just about covers some minimal basics - if I make any knowledge assumptions, please let me know and I'll elaborate


I'm going to talk about bluff bodies for the most part as that's what our cars are (with exceptions - basjoos!).
So lets look at some simple objects and what happens....
A cylinder is a classic example:

So, the flow in the front is generally nice, even and laminar. But downstream, there's some nasty eddies. The large vorticies off of a cylinder have their own natural frequency dependent on fluid velocity and cylinder diameter - coming at regular intervals. These remove energy from the body. The wake of a body typically has this same eddying motion, but not necessarily at a consistent frequency.
This is actually a VERY important phenomena as if this frequency matches the natural frequency of the cylinder (think a street lamp pole), the cylinder will vibrate divergently and without control, eventually self destruct. This is why many light poles, especially in wind prone areas, are tapered. Additionally, power lines may have spoilers and damping mechanisms attached. Even large sky scrapers have vibration control for wind induced motion - one method uses VERY heavy weights (think tons) on the top floor of the building; given a certain wind speed, oil is pumped under the weights so they can move (constrained and attached to the structure by springs)...
How about a sphere?
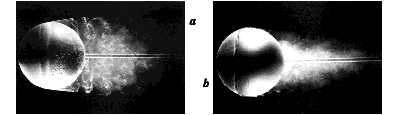
The sphere on the left (a) shows what happens with laminar flow. As soon as the pressure drop becomes significant you get separation, bugger.
So what's the deal with the picture on the right? How did we delay separation? That sphere has a trip wire. What does a trip wire do? It perturbs the boundary layer, a lot. Enough so that nearly the entire boundary layer in contact with the sphere is turbulent.
So how does this change cD? Here's some graphics:
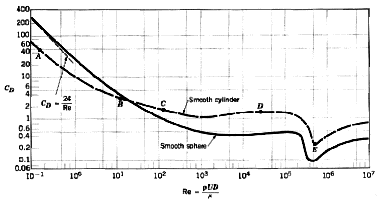
cD versus Reynolds #
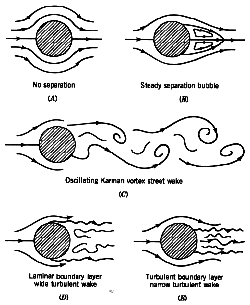
Points on the graph above correspond to the letters below each case.
"Whoa Whoa Whoa!! Are you telling me cD changes with velocity?"
Yes, yes I am - but don't get too excited as this mainly applies to small objects. cD drops significantly as the Re# increases. BUT, it remains nearly constant for large Re#'s in the laminar region and approaches a constant value after the transitional region. As a car is very wide compared to something like a tennis ball, the Reynolds number is high - very high in fact (on order of 10^6 @ 55mph and 10^5 at ~3mph).
Balls!
Sports balls, believe it or not, have some great design... Smooth balls are not very efficient aerodynamically. This is why golf balls have dimples, tennis balls have fuzz, cricket balls have a stubby seam, baseballs have stitches, American footballs are textured, soccer balls have seams (Everywhere). Okay, some of this is traditional - but it still plays a role.

Roughness and cD... Having a bit of roughness induces turbulence. Look at the photo above of the sphere with the trip wire - surface roughness is very similar, in effect, to a trip wire.
This results in a reduction of cD as shown in the above graph (which just so happens to also be in my fluid mechanics book - Fundamentals of Fluid Mechanics produced by John Wiley & Sons

). Relative roughness is t/D, t being the thickness of "rough" and D being the diameter of a sphere/cylinder. The dashed line on the left is a golf ball.
And on the subject of
golf balls... why? The dimples! Why? The dimples act, in effect, like a trip wire. They promote a transition to turbulent flow. By doing so, we get higher skin friction (yet another name for viscous losses). BUT, remember the definition of a bluff body? A golf ball is a sphere - and spheres are bluff bodies. Most of their losses come from the wake - so if we can move the wake further back, we can reduce the wake size and reduce the losses from the wake. It just so happens that by increasing the turbulence, you decrease your drag by reducing where most of the drag is coming from - flow separation (wake).
------
Okay, so what's all of this talk about the benefits of turbulent flow? Turbulence is the most misunderstood concept with regards to aerodynamics, in my opinion. Nature has known for millions of years of the benefits of turbulence. This is why dermal denticles on sharks help lower their cD. Likewise the bumpy tubercles of a whale fin. etc.

The key difference between induced turbulence and the turbulence as a result of a shape is the size. Larger eddies, swirls, etc. contain more energy than smaller ones. So if we induce smaller eddies, with a small amount of energy - we move into the turbulent regime without a whole lot of loss that you'd typically associate with the word "turbulence". This will typically be referred to as "energizing the boundary layer." This can happen on a very VERY small scale - the smallest of which is the
Kolmogorov micro scale. <-- keep in mind that the mathematic description of turbulence is not solved - we just can't do it, yet

Some very notable physicist when asked what they would like to ask God have replied:
Werner Heisenberg: "When I meet God, I am going to ask him two questions: Why relativity? And why turbulence? I really believe he will have an answer for the first."
Horace Lamb: "I am an old man now, and when I die and go to heaven there are two matters on which I hope for enlightenment. One is quantum electrodynamics, and the other is the turbulent motion of fluids. And about the former I am rather optimistic."
^^both of these were mentioned in my lectures

--------
Vortex Generators.....
Okay, so I've been building up to this as it has come up a lot recently - trying to give some background before I get to it.
Here's the answer to some straightforward (I hope) questions:
1. Does it increase turbulence?
Yes - the idea is, move the wake back with turbulent flow
2. Does it reduce cD?
The devil's in the details. Properly applied, absolutely yes. Nature has proven this through millions of years of evolutionary change. CFD and tunnel testing too

3. wtf counts as a vortex generator?
Almost anything that perturbs flow - from commercially sold tabs to dimples to stiff fuzz (think tennis ball). Vortex Generator does not necessarily refer to one particular product (nor do I endorse any such product).
4. How much improvement?
See #2 - Mitsubishi has published a paper reporting a cD reduction of 6 points (.006) on a Lancer which can be considered significant in the cD world. They used delta wing type vortex generators placed 100mm in front of the predicted separation point:


Note the smaller wake size and how the separation point is further down the rear glass:

Whew, freeking long.... Questions/additions? Let me know if I missed a typo too
